前段时间在开发抽奖游戏,需要生成一个随机数。游戏的规则之一是范围在 [1, 5] 的随机数是肯定不中奖的数字,对应的核心实现就是生成一个范围在 [1, 5] 的随机数。这是一个非常简单的实现,我也很果断地写下了这段代码:
return Math.random() * 5 + 1;return Math.random() * 5 + 1;然而这个接口返回的不是整数,不符合要求,所以被改成了这样:
return Math.ceil(Math.random() * 5 + 1);return Math.ceil(Math.random() * 5 + 1);于是悲剧就这样发生了 ~~
本来这个接口生成的随机数是范围 [1, 6) 的任意小数,而预期的是范围 [1, 5] 的任意整数,结果由于 Math.ceil(),实际输出的是范围 [1, 6] 的整数,其中整数 6 却是一个可能中奖的数字。最终,结局就是 ~~~~
Math.ceil(x) v.s. Math.floor(x) v.s. Math.round(x)
The Math.ceil() function returns the smallest integer greater than or equal to a given number. The Math.floor() function returns the largest integer less than or equal to a given number.
Math.ceil() 方法对于小数的取舍策略是向上取整,而 Math.floor() 则是向下取整,相当于去掉小数部分。例如,对于 Math.random() * 5 + 1 生成的一个随机数 5.650360632222146,使用 Math.ceil() 方法所得到的结果是 6,而 Math.floor() 方法所得到的结果是 5。显然后者的结果才是符合范围 [1, 5] 的要求。Mozilla Developer Network 给出了一个标准的生成一定范围内的随机整数的 代码示例 :
// Returns a random integer between min (included) and max (included)
// Using Math.round() will give you a non-uniform distribution!
function getRandomIntInclusive(min, max) {
return Math.floor(
Math.random() * (max - min + 1)
) + min;
}// Returns a random integer between min (included) and max (included)
// Using Math.round() will give you a non-uniform distribution!
function getRandomIntInclusive(min, max) {
return Math.floor(
Math.random() * (max - min + 1)
) + min;
}在注释里提到了 Math.round() 方法。该方法就是用 四舍五入 对小数进行取舍。Mozilla Developer Network 的描述如下:
If the fractional portion of number is 0.5 or greater , the argument is rounded to the next higher integer. If the fractional portion of number is less than 0.5 , the argument is rounded to the next lower integer.
如果 number 的小数部分是 .5 或者更大,输入的参数被四舍五入到下一个的更大的整数。 如果 number 的小数部分小于 .5,输入参数被四舍五入到下一个更小的整数。
所以,正如注释所说,在生成一定范围内的随机整数,如果 使用 Math.round() 会产生一个非均匀分布的随机数 。
parseInt(string, radix)
其实也可以直接使用 parseInt 函数来舍去小数部分。按照 ECMAScript Language Specification 对该函数的 描述 :
parseInt may interpret only a leading portion of string as an integer value; it ignores any characters that cannot be interpreted as part of the notation of an integer, and no indication is given that any such characters were ignored.
parseInt 函数可以只把 string 的开头部分解释为整数值;它会忽略所有不能解释为整数的字符,对于这些被忽略的字符,不会给出任何提示。
因此 parseInt 函数会忽视非整型数字的字符且遇到这样的字符就会停止解析。按道理可以利用这个特性来移除数字的小数部分,实现形似 Math.floor() 方法的效果。不过这仅仅只是个人猜测,我还是搜了一下 V8 对 parseInt 方法的实现:
// ECMA-262 - 15.1.2.2
function GlobalParseInt(string, radix) {
if (IS_UNDEFINED(radix) || radix === 10 || radix === 0) {
// Some people use parseInt instead of Math.floor. This
// optimization makes parseInt on a Smi 12 times faster (60ns
// vs 800ns). The following optimization makes parseInt on a
// non-Smi number 9 times faster (230ns vs 2070ns). Together
// they make parseInt on a string 1.4% slower (274ns vs 270ns).
if (%_IsSmi(string)) return string;
if (IS_NUMBER(string) &&
((0.01 < string && string < 1e9) ||
(-1e9 < string && string < -0.01))) {
// Truncate number.
return string | 0;
}
string = TO_STRING_INLINE(string);
radix = radix | 0;
}
}// ECMA-262 - 15.1.2.2
function GlobalParseInt(string, radix) {
if (IS_UNDEFINED(radix) || radix === 10 || radix === 0) {
// Some people use parseInt instead of Math.floor. This
// optimization makes parseInt on a Smi 12 times faster (60ns
// vs 800ns). The following optimization makes parseInt on a
// non-Smi number 9 times faster (230ns vs 2070ns). Together
// they make parseInt on a string 1.4% slower (274ns vs 270ns).
if (%_IsSmi(string)) return string;
if (IS_NUMBER(string) &&
((0.01 < string && string < 1e9) ||
(-1e9 < string && string < -0.01))) {
// Truncate number.
return string | 0;
}
string = TO_STRING_INLINE(string);
radix = radix | 0;
}
}Smi: Smi represents integer Numbers that can be stored in 31 bits.
按照注释,确实存在使用 parseInt 来代替 Math.floor() 的做法。另外,V8 还为此做了性能优化(可惜结果牺牲了解释字符串的性能,哈哈 ~~ )从这段注释也说明了, 使用 parseInt 函数的效果与 Math.floor() 一致 。
进一步阅读 V8 对 parseInt 函数的实现代码,可以看到,移除小数点部分的实现方法是 位逻辑运算 。这里的 parseInt 函数使用了或运算(return string | 0)来去除小数部分。
位逻辑运算
位逻辑运算是位运算的一种。至于位运算,它是一种非常底层的运算,直接处理每一个比特位,速度快但不直观。我们知道,JavaScript 的数值都是以 64 位浮点数的形式进行存储的,但是位运算只作用于 32 位整数,所以,当进行位运算时,JavaScript 会把数值自动转换成带符号的 32 位整数进行运算,结果相当于丢弃小数部分,返回整数部分(这个整数是带有符号的 32 位整数)。因此我们可以利用这样的特性来实现类似 Math.ceil() 和 Math.floor() 方法。
或运算符 OR
或运算的符号为 |,其真值表如下:
| a | b | a OR b |
|---|---|---|
| 1 | 0 | 1 |
| 1 | 1 | 1 |
| 0 | 0 | 0 |
| 0 | 1 | 1 |
按照或运算的规则,当一个数与 0 进行或运算,那么结果就相当于剔除小数部分,整数部分不变。例如,对于小数 6.1923 进行位运算,首先会舍去小数部分,而整数部分 6 的二进制表示为 110,接着与 0 进行或运算,其结果依然为 110,对应的十进制表示就是 6。不管对于正数还是负数,使用或运算来保留整数部分、移除小数部分都是有效的。
否运算 NOT
否运算的符号为 ~,其真值表如下:
| a | NOT a |
|---|---|
| 1 | 0 |
| 0 | 1 |
按照否运算的规则,一个数对自身进行连续两次否运算,其结果还是自身。所以我们可以利用这个规则以及位运算只作用于整数的特性,对一个小数进行连续两次否运算,就可以去除小数部分了。例如对 5.645 进行两次否运算 ~~(5.545) 其运算结果就是 5。
异或运算 XOR
异或运算的符号为 ^,其真值表如下:
| a | b | a XOR b |
|---|---|---|
| 1 | 0 | 1 |
| 1 | 1 | 0 |
| 0 | 0 | 0 |
| 0 | 1 | 1 |
按照异或的运算规则,当一个数与 0 进行异或后,其结果与进行或运算的效果一样,都是相当于剔除小数部分,整数部分不变。
移位运算符
我们还可以运用移位运算符,因为它跟位逻辑运算符同为位运算符,遵循同样的特性,只是这次我们位移 0 个比特位。
JavaScript 的移位运算符有三种: 左移运算 ,它的符号是 <<,表示把一个数的二进制形式向左边移动,尾部补 0; 右移运算 ,它的符号是 >>,表示把一个数的二进制形式向右边移动,符号位保持不变,最左边补上二进制 0;无符号的右移运算符( Zero-fill Right Shift ),它的符号是 >>>,表示把一个数的二进制位形式向右移动,同时符号位总是用二进制 0 填充,所以结果总是非负的,即使右移 0 个比特位,结果同样也是非负的。
不过我们只是想要去除小数位,但不想移动整数部分的二进制,所以这次我们就只是简单地利用了位运算的特性,直接对一个小数的整数部分移动 0 位,这样就可以去除小数部分,也保持了整数部分的值了。例如 5.454 << 0 === 5 或 6.454 >> 0 === 6 或 7.342 >>> 0 === 7。
特别需要注意的是,对于移除小数部分, 仅有左移运算符和右移运算符对正数和负数都是有效的 ,而无符号的右移运算符只对正数有效,对负数则是无效的,这是因为该运算是无符号运算,其结果是一个无符号的 32 位整型。举个例子,对于数 -1,在 JavaScript 的存储形式为 -1 的补码,即 11111111 11111111 11111111 11111111,进行 0 比特位的无符号右移运算后,最左边不再充当符号位而是普通的数值,因此,其十进制结果就是 2^32 - 1 = 4294967295。
Math.trunc(x)
Math.trunc(x) 方法是 ECMAScript 6 新增的方法,用于获取一个数的整数部分。Mozilla Developer Network 给出的描述如下:
Unlike other three Math methods: Math.floor(), Math.ceil() and Math.round(), the way Math.trunc() works is very simple and straightforward, just truncate the dot and the digits behind it, no matter whether the argument is a positive number or a negative number.
不同于其他的三个 Math 方法:Math.floor(),Math.ceil() 和 Math.round();Math.trunc() 的执行逻辑非常简单,不管是正数还是负数,都只删除数字的小数点以及小数部分。
Mozilla Developer Network 给出了 Math.trunc() 方法的一个 polyfill 实现:
Math.trunc = Math.trunc || function(x) {
return x < 0 ? Math.ceil(x) : Math.floor(x);
};Math.trunc = Math.trunc || function(x) {
return x < 0 ? Math.ceil(x) : Math.floor(x);
}; 这里之所以要处理 x < 0 的情况是因为去掉负的小数部分的结果与去掉正的小数的结果相反:对正的小数而言,去掉小数点意味着缩小小数;对于负的小数而言,去掉小数点则意味着扩大小数。通过以下两张对数学运算符 floor() 与 ceil() 的描述可以明显看出: 对负的小数使用 ceil(),对正的小数使用 floor() ,保证去掉小数部分的同时,保持整数部分不变:
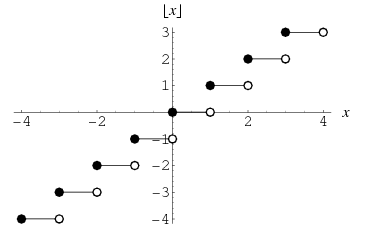
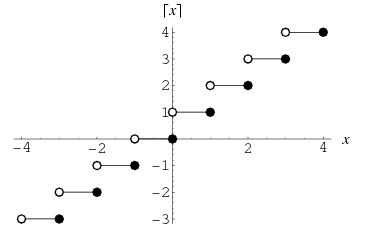
另外一个问题来了:Math.trunc() 是不是与 parseInt 函数或者 Math.floor() 的实现逻辑一样的呢?对于这个问题,我们只能继续扒 V8 的源代码了。以下是 V8 对 Math.trunc() 的实现:
// ES6 draft 09-27-13, section 20.2.2.34.
function MathTrunc(x) {
x = +x;
if (x > 0) return %_MathFloor(x);
if (x < 0) return -%_MathFloor(-x);
// -0, 0 or NaN.
return x;
}// ES6 draft 09-27-13, section 20.2.2.34.
function MathTrunc(x) {
x = +x;
if (x > 0) return %_MathFloor(x);
if (x < 0) return -%_MathFloor(-x);
// -0, 0 or NaN.
return x;
}似乎源码的实现与上述的 JavaScript polyfill 很像!
通过查阅 ECMAScript Language Specification ,可以找到对 Math.ceil() 方法的 描述 :The value of Math.ceil(x) is the same as the value of -Math.floor(-x). Math.ceil(x) 的值与 -Math.floor(-x) 的值一致__,另外对 Math.floor() 方法的 描述 则有:The value of Math.floor(x) is the same as the value of -Math.ceil(-x). Math.floor(x) 的值与 -Math.ceil(-x) 的值一致。对应到源码的实现则分别为:
// ECMA 262 - 15.8.2.6
function MathCeil(x) {
return -%_MathFloor(-x);
}
// ECMA 262 - 15.8.2.9
function MathFloorJS(x) {
return %_MathFloor(+x);
}// ECMA 262 - 15.8.2.6
function MathCeil(x) {
return -%_MathFloor(-x);
}
// ECMA 262 - 15.8.2.9
function MathFloorJS(x) {
return %_MathFloor(+x);
}到这里,也就清楚 Math.trunc() 的 JavaScript polyfill 实现与 V8 的实现其实如出一辙。
总结
对于从一定区间内随机抽取一个数,一般都会调用 Math.random() 方法,不过该方法总是返回一个小数,所以存在超过最大值(或者最小值)的可能,因此我们往往需要裁掉小数部分,只保留整数部分。处理的方法有三种:
- 对正的小数使用 Math.floor() ,对负的小数使用 Math.ceil();
- 使用 Math.trunc();
- 使用”或运算“。